PAT（甲級）2019年冬季考試 7-4 Cartesian Tree

7-4 Cartesian Tree (30分)

A Cartesian tree is a binary tree constructed from a sequence of distinct numbers. The tree is heap-ordered, and an inorder traversal returns the original sequence. For example, given the sequence { 8, 15, 3, 4, 1, 5, 12, 10, 18, 6 }, the min-heap Cartesian tree is shown by the figure.

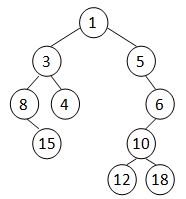
Your job is to output the level-order traversal sequence of the min-heap Cartesian tree.

Input Specification:

Each input file contains one test case. Each case starts from giving a positive integer N (≤30), and then N distinct numbers in the next line, separated by a space. All the numbers are in the range of int.

Output Specification:

For each test case, print in a line the level-order traversal sequence of the min-heap Cartesian tree. All the numbers in a line must be separated by exactly one space, and there must be no extra space at the beginning or the end of the line.

Sample Input:

10
8 15 3 4 1 5 12 10 18 6`

Sample Output:

1 3 5 8 4 6 15 10 12 18

題目限制：

題目大意：

現給定一顆笛卡爾樹的中序序列，它知足小根堆的性質，如今須要輸出其層序遍歷。

算法思路：

大體思路就是建樹和層序遍歷，其惟一的難點在於建樹，其實只要知道了根節點的位置就不是問題，首先咱們如今有這顆樹的中序序列，保存在origin數組中，同時使用unordered_map<int,int> pos 保存每個節點在中序序列中的位置，建樹的關鍵是得只要每個子樹的根節點和在中序遍歷中的位置，這裏的子樹都知足小根堆的性質，說明在[inL,inR]之間最小的數字就是當前子樹的根節點，那麼咱們使用getMin得到original中的[left,right]最小的那個數字，代碼以下：

// 在original的[left,right]中找到最小的那個數字
int getMin(int left,int right){
    int Min = 0x3fffffff;
    for(int i=left;i<=right;++i){
        Min = Min>original[i]?original[i]:Min;
    }
    return Min;
}

接下來就能夠使用createTree函數來進行建樹了，代碼以下：

Node* createTree(int inL,int inR){
    if(inL>inR) return nullptr;
    Node* root = new Node;
    root->data = getMin(inL,inR);
    int k = pos[root->data];// 根節點的位置 
    //[inL,k-1]爲左子樹
    root->left = createTree(inL,k-1);
    //[k+1,inR]爲右子樹
    root->right = createTree(k+1,inR);
    return root;
}

最後就是層序遍歷並輸出。

注意點：

• 一、結點數值有點偏大，使用數組保存結點的位置會致使段錯誤，使用map比較好

提交結果：

AC代碼：

#include<cstdio>
#include<queue>
#include<unordered_map>

using namespace std;

struct Node{
    int data;
    Node *left;
    Node *right;
};

int original[40];
unordered_map<int,int> pos;// 每個節點在中序序列中的位置

// 在original的[left,right]中找到最小的那個數字
int getMin(int left,int right){
    int Min = 0x3fffffff;
    for(int i=left;i<=right;++i){
        Min = Min>original[i]?original[i]:Min;
    }
    return Min;
}

Node* createTree(int inL,int inR){
    if(inL>inR) return nullptr;
    Node* root = new Node;
    root->data = getMin(inL,inR);
    int k = pos[root->data];// 根節點的位置 
    //[inL,k-1]爲左子樹
    root->left = createTree(inL,k-1);
    //[k+1,inR]爲右子樹
    root->right = createTree(k+1,inR);
    return root;
}

int num = 0;
void BFS(Node* root){
    queue<Node*> q;
    q.push(root);
    while (!q.empty()){
        Node* t = q.front();
        q.pop();
        if(num==0){
            printf("%d",t->data);
            ++num;
        } else {
            printf(" %d",t->data);
        }
        if(t->left){
            q.push(t->left);
        }
        if(t->right){
            q.push(t->right);
        }
    }
}

int main(){
    int N;
    scanf("%d",&N);
    for (int i = 0; i < N; ++i) {
        scanf("%d",&original[i]);
        pos[original[i]] = i;
    }
    Node* root = createTree(0,N-1);
    BFS(root);
    return 0;
}